Updated Jun 26, 2020 Indifference curves are graphs that represent various combinations of two commodities which an individual considers equally valuable. The axes of those graphs represent one commodity each (e.g., good A and good B). Indifference curves are widely used in microeconomics to analyze consumer preferences, the effects of subsidies and taxes, and a few other concepts. Although they come in many shapes and sizes, most of them share a few important properties. Thus, we will look at the four most important properties of indifference curves in more detail below. Virtually all indifference curves have a negative slope. That is, they slope downward from left to right. The slope of the curve shows the rate of substitution between two goods, i.e. the rate at which an individual is willing to give up some quantity of good A to get more of good B. If we assume that the individual likes both goods, the quantity of good B has to increase as the quantity of good A decreases, to keep the overall level of satisfaction the same. Because both axes each represent one of the two goods, this relationship results in a downward sloping curve. This becomes pretty obvious if we look at the indifference map below. Consumers will always prefer a higher indifference curve to a lower one. This is due to the basic economic assumption that “more is always better“. Think about it if someone were to ask you if you wanted a free slice of pizza or an entire pizza for free, what would you say? Who says no to free pizza, right? Now, of course, it’s not always that simple, but in basic economic theory, we can assume that consumers have a preference for larger quantities. This is reflected graphically in the indifference map. The higher the indifference curves are, the larger the quantities of both goods. And thus, the more preferable the curve becomes.1. Indifference Curves are Downward Sloping
2. Higher Indifference Curves Are Preferred to Lower Ones
3. Indifference Curves Cannot Intersect
Two indifference curves can’t cross. To understand why this is the case, we can look at what would happen if they did intersect. As we know, all combinations of good A and good B that lie on the same indifference curve make the consumer equally happy. Therefore, if two indifference curves were to cross, they would both have to provide the consumer with the same level of total satisfaction, because the exact point where they intersect (i.e., point A) is on both curves. Thus, all other combinations on both curves would have to provide the same level of satisfaction as well. However, if we compare point B and point C, we can see that point C offers more of good A and good B (90 and 140) as compared to point B (80 and 130). As we already learned above, consumers always prefer larger quantities. Therefore both curves can’t provide the same level of satisfaction, which means they can never intersect.
4. Indifference Curves are convex (i.e., bowed inward)
In most cases, indifference curves are bowed inward. This has to do with the marginal rate of substitution (MRS). We know that the marginal utility of consuming a good decreases as its supply increases (see also diminishing marginal utility). Therefore consumers are willing to give up more of this good to get another good of which they have little. Let’s look at the graph below to illustrate this. If a consumer has a lot of good B, the MRS is 3 units of good B per unit of good A. If she has more of good A, the MRS is 0.5 units of good B per unit of good A. In other words, if they have a lot of good B, they are more willing to trade some of it in to get an additional unit of good A and vice versa. Because of this relationship, the indifference curve is bowed inward (i.e., convex).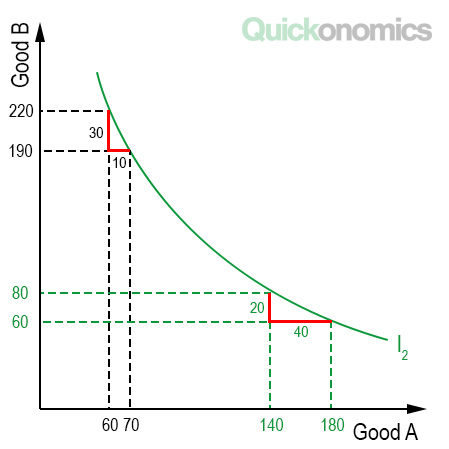
In a Nutshell
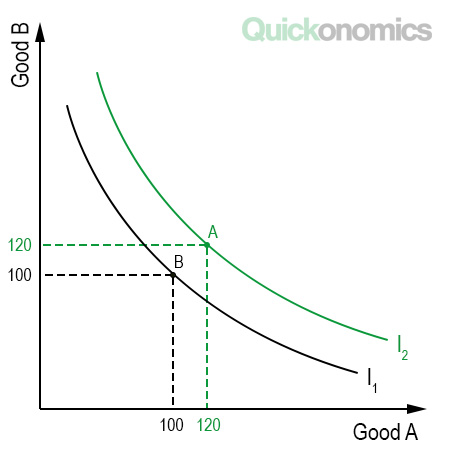
Indifference curves are graphical representations of various combinations of two commodities which an individual considers equally valuable. They are used to analyze consumer preferences and a number of other concepts. There are four important properties of indifference curves that describe most of them: (1) They are downward sloping, (2) higher indifference curves are preferred to lower ones, (3) they cannot intersect, and (4) indifference curves are convex (i.e. bowed inward).